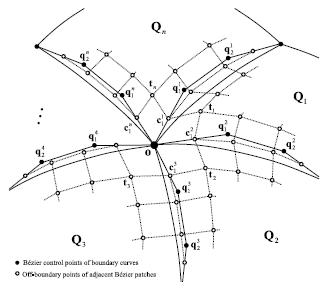
We propose a local method of constructing piecewise G1 Bezier patches to span an irregular curve network, without modifying the given curves at odd- and 4-valent node points. Topologically irregular regions of the network are approximated by implicit surfaces, which are used to generate split curves, which subdivide the regions into triangular and/or rectangular sub-regions. The subdivided regions are then interpolated with Bezier patches. We analyze various singular cases of the G1 condition that is to be met by the interpolation and propose a new G1 continuity condition using linear and quartic scalar weight functions. Using this condition, a curve network can be interpolated without modification at 4-valent nodes with two collinear tangent vectors, even in the presence of singularities. We demonstrate our approach in a ship hull.-Interpolating G1 Bezier surfaces over irregular curve networks for ship hull design, Doo-Yeoun Cho a, Kyu-Yeul Lee b, Tae-Wan Kim, Computer-Aided Design 38 (2006) 641.660